Bordered Heegaard Floer homology seminar

Fridays 3 PM in MS 5138

List of talks

1. Date: January 13, 2023
   Title: A_infinity structures
   Speaker: Advika Rajapakse
   Abstract: We will review the definitions of A∞-algebras, A∞-modules, and tensor products of A∞-modules, before reviewing basic properties of A∞ tensor products. We will then define type D structures on graded k-modules and show how type D homomorphisms can be identified with A∞ homomorphisms.
   Notes:A_infinity Structures Notes
   
   
2. Date: January 20, 2023
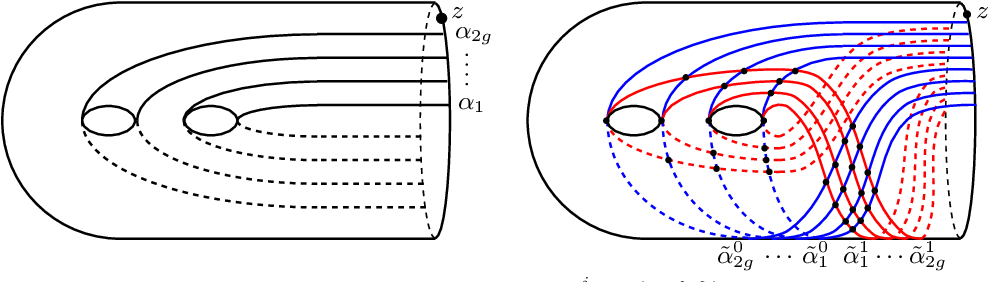
   Title: The algebra associated to a pointed mathched circle
   Speaker: Colin Ni
   Abstract: We will discuss the differential graded algebra A(Z) of a so-called pointed matched circle of a closed oriented 2-manifold. In particular, we will carefully compute A(Z) in the case of a torus. Later in this seminar, we will see that the bordered Heegaard Floer invariants CFD and CFA are respectively a left differential module over A(-Z) and a right A_infty module over A(Z).
   Notes:Matched Circle Notes
   
   
3. Date: January 27, 2023
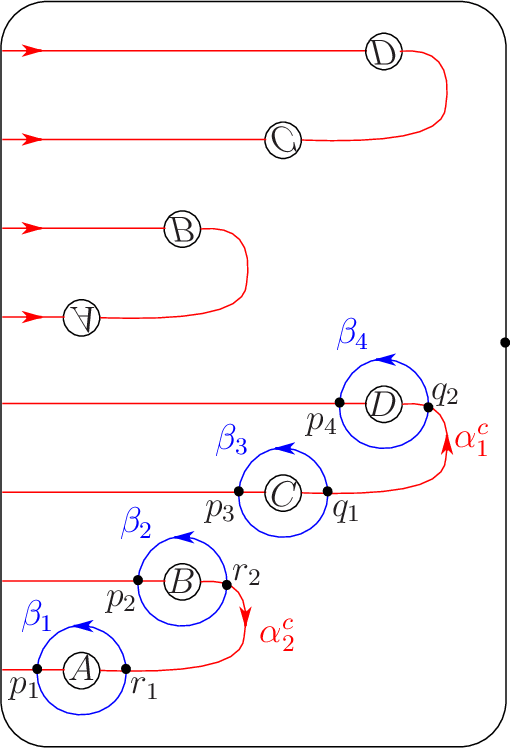
   Title: Bordered Heegaard diagrams
   Speaker: Jung Joo Suh
   Abstract: In this talk, we explore the connections between bordered 3-manifolds, which are compact 3-manifolds with boundary with extra data about the their boundaries, and bordered Heegard diagrams, which are compact surfaces with boundary, together with extra information. Specifically, we will show how to construct a bordered 3-manifold from a bordered Heegard diagrams, and how they help characterize said bordered 3-manifold. In addition, we will discuss some connections to Morse Theory, and describe spin^c structures associated with bordered Heegard diagrams.
   
   
4. Date: February 3, 2023
   Title: Moduli spaces
   Speaker: Roman Krutowski
   Abstract: The key tool for defining algebraic structures in any Floer-type theory is the count of certain J-holomorphic curves. In this talk, we will describe the moduli spaces of such curves in a "strip" over a given surface with boundary and compactifications of such spaces given by holomorphic combs. Time permitting, we will discuss some gluing results essential for the Bordered Heegaard Floer homology.
   
   
5. Date: February 10, 2023
   Title: Type D modules
   Speaker: Yan Tao
   Abstract: In this talk, we will define the type D module CFD of a bordered Heegaard diagram. Since it will be convenient to choose certain structures in this definition, we will focus on showing that CFD does not depend on this choice, and moreover that CFD is a bordered 3-manifold invariant. Time permitting, we will also give a version of CFD with twisted coefficients.
   
   
6. Date: February 17, 2023
   Title: Type A modules
   Speaker: Yan Tao
   Abstract: In this talk, we will define the type A module CFA of a bordered Heegaard diagram. In contrast to the type D module CFD from last week's talk, we will show that CFA is an A-infinity module. Similarly to CFD, we will then show that CFA does not depend on the choice of almost complex structure used to define the moduli spaces and that CFA is a bordered 3-manifold invariant.
   
   
7. Date: February 24, 2023
   Title: Pairing theorem
   Speaker: David Popovic
   Abstract: In this talk, we will introduce the pairing theorem, connecting CFD and CFA structures with the classical Heegaard Floer homology. We will give an outline of the proof of the pairing theorem and a few example calculations.
   
   
8. Date: March 3, 2023
   Title: Immersed curves: setup
   Speaker: Eilon Reisin-Tzur
   Abstract: TBD
   
   
9. Date: March 10, 2023
   Title: Immersed curves: applications
   Speaker: Konstantinos Varvarezos
   Abstract: TBD
   
   
10. Date: March 17, 2023
    Title: TBD
    Speaker: Raphael Rouquier
    Abstract: TBD