Problem 1
Let
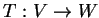
be a linear transformation.
a) Let
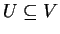 be a subspace of
be a subspace of 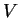 . Show that
. Show that
 is a subspace of
is a subspace of 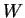 . If
. If 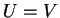 , then
, then 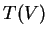 is the subspace called
the range of the linear transformation
is the subspace called
the range of the linear transformation  .
.
b) Let
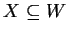 be a subspace of
be a subspace of 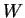 . Show that
. Show that
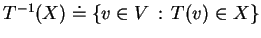 is a subspace of
is a subspace of 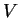 . If
. If  , then
, then
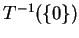 is the
subspace called the null space of
is the
subspace called the null space of 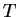 .
.
Problem 2
Let
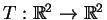
be the reflection through the
line
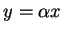
on the plane. Find the matrix of this transformation
with respect to the standard basis.
Problem 3
Recall the following facts:
- Two non-zero vectors in
 are perpendicular iff their dot
product is
are perpendicular iff their dot
product is 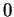 ;
;
- The cross product of two linearly independent vectors in
 is perpendicular to the plane spanned by those vectors in
is perpendicular to the plane spanned by those vectors in
 .
.
Using these facts, solve the following problem: Let
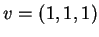
,
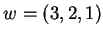
and
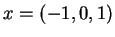
be vectors in

. Let
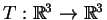
be the linear transformation defined as the composition of the following:
1) first, perform rotation in the plane perpendicular to

by angle
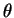
;
2) then, do a flip in the plane perpendicular to
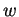
;
3) finally, do a (counterclockwise) rotation in the plane perpendicular to
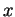
by angle
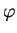
;
Determine the matrix of this linear transformation in the standard basis in

. (Do not multiply the three matrices you will have).
Problem 4
Let
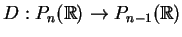
be the differentiation
transformation defined by
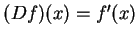
and
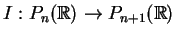
be the integration transformation defined by
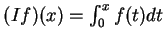
.
Find the matrices of these transformations with respect to the standard bases
in
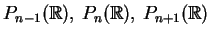
.
Problem 5
Let
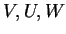
be vector spaces such that
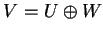
. Let
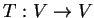
be a linear transformation such that
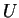
is invariant under
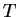
. Is
it true that
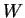
is also invariant under
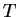
? Prove or give a counterexample.
![]() be a subspace of
be a subspace of ![]() . Show that
. Show that
![]() is a subspace of
is a subspace of ![]() . If
. If ![]() , then
, then ![]() is the subspace called
the range of the linear transformation
is the subspace called
the range of the linear transformation ![]() .
.
![]() be a subspace of
be a subspace of ![]() . Show that
. Show that
![]() is a subspace of
is a subspace of ![]() . If
. If ![]() , then
, then
![]() is the
subspace called the null space of
is the
subspace called the null space of ![]() .
.
 are perpendicular iff their dot
product is
are perpendicular iff their dot
product is 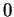 ;
;
 is perpendicular to the plane spanned by those vectors in
is perpendicular to the plane spanned by those vectors in
 .
.